基于非参数估计方法的违约回收率密度函数估计研究
浙江财经学院硕士学位论文I摘要对违约概率的研究一直以来是学者关注的重点,模型随着假设条件从简单到贴近实际和关注角度的变化发展出结构模型、简约模型和信用VaR模型。这些模型的最终意图都是发展出一套科学系统的体系来度量或预测违约概率,以求合理定价或防范未来可能发生的损失。而对于另一项风险因子违约损失率,大都认为其属于统计范畴而少有重视,直到近些年,不良资产的增加违约事件的增多才带动一些学者和机构对损失率的研究。这类研究基本涉及统计方法、分布形态、影响因素等方面,其中在分布形态研究上,目前主要分为两类:一类是利用直方图等统计手段观察分布形态,计算均值和方差;一类是使用回收率样本做某种分布函数的参数估...
相关推荐
-
七年级数学下册(易错30题专练)(沪教版)-第13章 相交线 平行线(原卷版)VIP免费

 2024-10-14 25
2024-10-14 25 -
七年级数学下册(易错30题专练)(沪教版)-第13章 相交线 平行线(解析版)VIP免费

 2024-10-14 28
2024-10-14 28 -
七年级数学下册(易错30题专练)(沪教版)-第12章 实数(原卷版)VIP免费

 2024-10-14 27
2024-10-14 27 -
七年级数学下册(易错30题专练)(沪教版)-第12章 实数(解析版)VIP免费

 2024-10-14 19
2024-10-14 19 -
七年级数学下册(压轴30题专练)(沪教版)-第15章平面直角坐标系(原卷版)VIP免费

 2024-10-14 19
2024-10-14 19 -
七年级数学下册(压轴30题专练)(沪教版)-第15章平面直角坐标系(解析版)VIP免费

 2024-10-14 27
2024-10-14 27 -
七年级数学下册(压轴30题专练)(沪教版)-第14章三角形(原卷版)VIP免费

 2024-10-14 19
2024-10-14 19 -
七年级数学下册(压轴30题专练)(沪教版)-第14章三角形(解析版)VIP免费
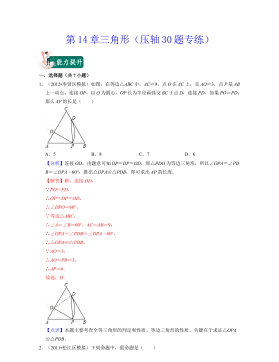
 2024-10-14 30
2024-10-14 30 -
七年级数学下册(压轴30题专练)(沪教版)-第13章 相交线 平行线(原卷版)VIP免费
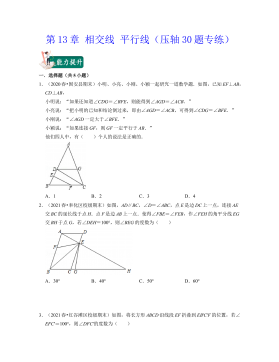
 2024-10-14 26
2024-10-14 26 -
七年级数学下册(压轴30题专练)(沪教版)-第13章 相交线 平行线(解析版)VIP免费

 2024-10-14 22
2024-10-14 22
相关内容
-

七年级数学下册(压轴30题专练)(沪教版)-第15章平面直角坐标系(解析版)
分类:中小学教育资料
时间:2024-10-14
标签:无
格式:DOCX
价格:15 积分
-

七年级数学下册(压轴30题专练)(沪教版)-第14章三角形(原卷版)
分类:中小学教育资料
时间:2024-10-14
标签:无
格式:DOCX
价格:15 积分
-

七年级数学下册(压轴30题专练)(沪教版)-第14章三角形(解析版)
分类:中小学教育资料
时间:2024-10-14
标签:无
格式:DOCX
价格:15 积分
-

七年级数学下册(压轴30题专练)(沪教版)-第13章 相交线 平行线(原卷版)
分类:中小学教育资料
时间:2024-10-14
标签:无
格式:DOCX
价格:15 积分
-

七年级数学下册(压轴30题专练)(沪教版)-第13章 相交线 平行线(解析版)
分类:中小学教育资料
时间:2024-10-14
标签:无
格式:DOCX
价格:15 积分






